Severe Weather

Hurricane energetics

Latent heat of water: the Fuel of Hurricanes

Hurricanes are extremely energetic storms with very warm cores, high winds and low central pressures. To fuel these storms, hurricanes must somehow tap into an enormous energy source. That energy source is the latent heat of water. We can estimate the amount of energy released by a hurricane knowing approximately how much rainfall a hurricane generated. Remember that for each gram of water vapor that is condensed, 590 calories of energy are released to heat the atmosphere. For comparison, TNT (Trinitrotoluene) releases 1000 cal/g in an explosion. So condensing a gram of water vapor to liquid at normal temperatures releases about 60% of the energy that detonating a gram of TNT would.

Now we want to take rainfall and estimate the latent heat energy released. Remember that 1 gram of liquid water takes up a volume of 1 cubic centimeter. So rainfall of 1 cm depth means that above every 1 square centimeter of surface, 1 cm of liquid water has condensed and fallen out. So in every 1 cm square air column, 590 calories of latent heat has been released.
Consider a 100 meter by 100 meter area that received 1 inch = 2.5 cm of rainfall. The area is 10,000 cm by 10,000 cm = 100,000,000 square centimeters. The total volume of condensed water that fell as rainfall was 100,000,000 square centimeter area x 2.5 cm depth = 250,000,000 cubic cm. Therfore the mass of water condensed 250,000,000 cubic cm x 1 gram/cubic cm = 250,000,000 grams. Therefore the total latent heat energy released into the air by the rainfall over the 100 meter by 100 meter area is 590 cal per gram of water x 250,000,000 gram of water = 150,000,000,000 calories = 150 billion calories.

So, to calculate the latent heat energy released into the air by a hurricane we need to know

1. the depth or amount of rainfall and
2. the area over which the rain fell along the track of the hurricane.

ENERGY RELEASED BY HURRICANE = total volume of rainfall x density of liquid water x latent heat release per gram water

The rainfall information we need can be estimated from satellite data. Katrina provides a nice example. Katrina was a very large and intense hurricane that released a huge amount of energy.

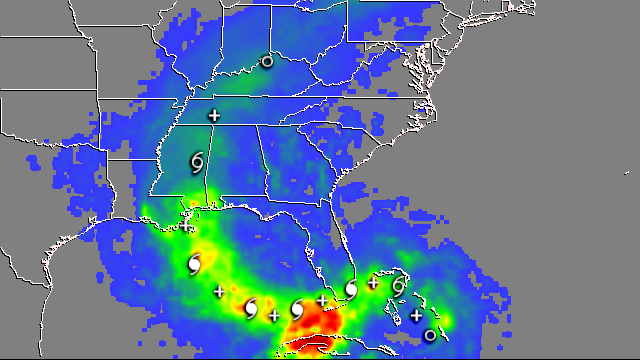Figure 3: Katrina rainfall

Movie of Katrina estimated rainfall from NASA (which shows color scale)

the rainfall produced by Katrina has been estimated from NASA's Tropical Rainfall Monitoring Mission (TRMM) as shown above. We can estimate the energy released by Katrina as follows: The swath of green (12-18 cm = 5-7 in of rainfall) is approximately 400 km wide and 1600 km long. The area is therefore 6x10¹⁵square centimeters. Multiplying the area by 15 cm of average rainfall gives the total rainfall volume of approximately 10¹⁷ cubic cm. Multiplying by 1 gram per cubic cm yields the total rainfall mass of 10¹⁷ grams. Multiplying by 590 cal/gm gives the approximate

TOTAL LATENT HEAT ENERGY RELEASED BY KATRINA = 6x10¹⁹ calories = 2.3x10¹⁷ btu (British Thermal Units) = 2.4x10²⁰ joules.

NOTE: Conversion between different energy units: 1 calorie = 4.184 joules = 0.004 btu.

Let's put this into perspective with some comparisons...
Power generator: Given that Katrina lasted about a week, its rate of energy production (= "power") over that week was approximately 3x10⁸ megawatts. The power generation of a typical nuclear generator is about 1,000 megawatts, or 300,000 times less than Katrina!
US annual power consumption vs. Katrina: We can also compare the energy released by Katrina with the entire annual energy consumption by the United States. According to the DOE figure below, the U.S. energy consumption for 2004 was 100,000,000,000,000,000 btu = 100 Quadrillion btu = 10¹⁷ btu, less than half of the energy Katrina released in a week.

Figure : US energy consumption from DOE 2004 Annual Energy Review

Comparison of Katrina's energy released (over a week) with energy released (instantaneously) in explosions:

Depth of warm water required to fuel a hurricane

The energy source for hurricanes is latent heat. This must come from evaporation off the ocean surface which will remove energy from the surface and therefore cool the ocean surface. We can see this effect in the following two images that show the sea surface temperature anomaly before and after Katrina came ashore. Anomaly means the difference between the actual sea surface temperature and the typical sea surface temperature for that date. It is clear that the passage of Katrina cooled the ocean surface temperature by approximately 2 °C.

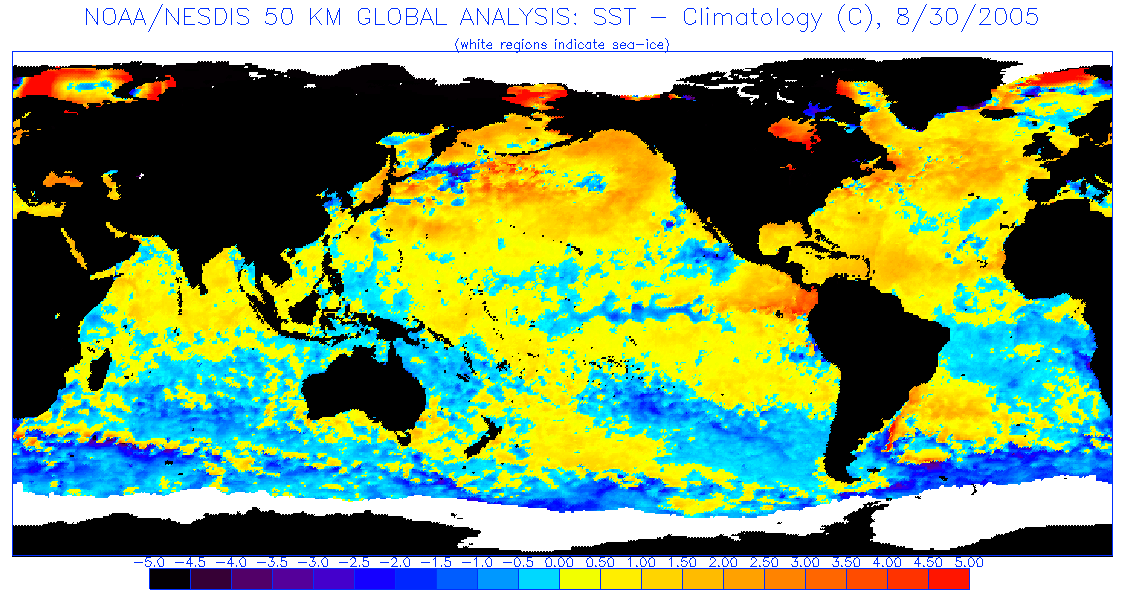
Figure: SST anomaly as Katrina came ashore along the Gulf coast

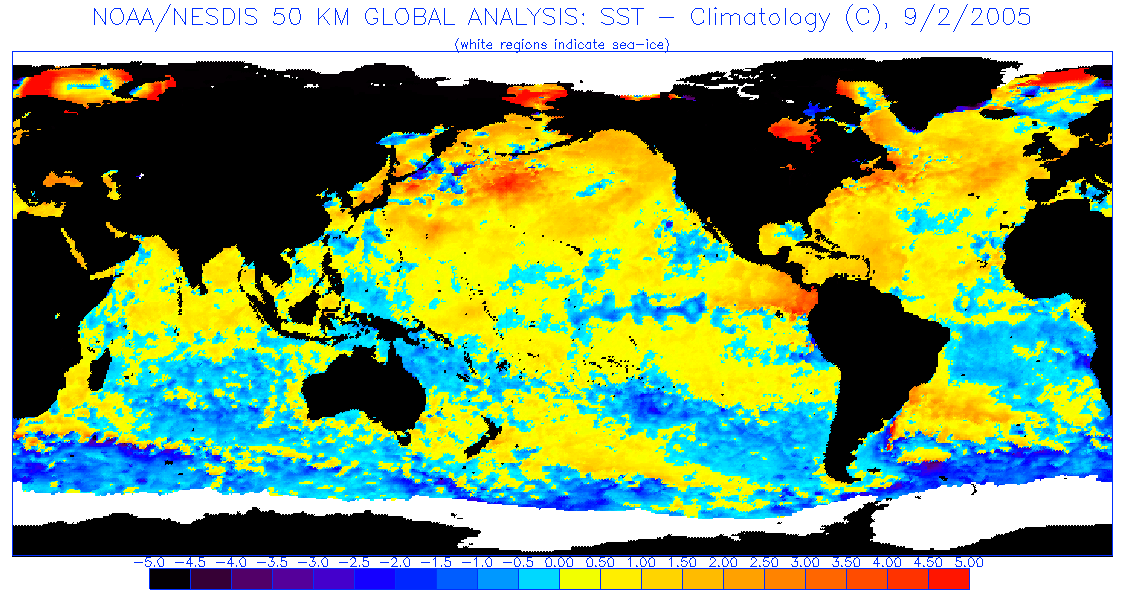
Figure: SST anomaly after Katrina came ashore (9/2/2005)

To understand this ocean cooling effect, recall an exercise we did earlier in class about the energy required to heat a gram of water ice until it was boiling. There were two rates of energy that are relevant here:

1. 1 calorie is added (lost) to raise (lower) the temperature of a gram of liquid water by 1°C
2. 590 calories are required to evaporate one gram of liquid water

So Katrina evaporatively removed approximately 9000 calories from each square centimeter of ocean surface along its path. The surface layer of the ocean is quite churned up by the hurricane's winds so the layer that is cooled extends to a significant depth. Let's try and figure out approximately what that depth is. So let's ask the question, if the surface layer of the ocean were cooled by 2°C by the evaporation, how deep would the cooled layer be? According to the energy transfer in item 1 above, cooling a gram of liquid water by 1°C causes the water to lose 1 calorie of energy. So if each gram of water in the layer cools by 2°C and therefore loses 2 calories, how many grams of water are needed to supply the 9000 calories of energy evaporatively removed from the surface? The answer is 9000/2 = 4500 grams per square centimeter of ocean surface.

So for each square cm of the ocean surface, 4500 grams of water must be cooled 2°C. Since the water has a density of 1 gm/cm³ and the surface area is 1 cm², the depth of the layer that is cooled by 2°C must be 4500 cm or 45 meters deep. Note that if the layer had cooled by only 1°C, the layer would have been twice as deep or 90 meters.

The crucial point is that it is NOT just the sea surface temperature that matters, it is the depth or thickness of the warm surface layer at the top of the ocean that stores the energy that fuels the hurricane intensity development and evolution. If the layer of warm water in front of the hurricane is shallow then the hurricane will weaken as it passes over that layer of water.

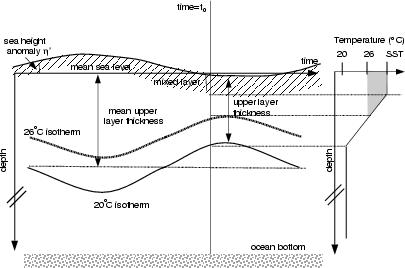
Figure: TCHP explanation cartoon

Tropical Cyclone Heat Potential (TCHP)

In an attempt to measure the amount of energy in the near surface ocean layer available to hurricanes, the Tropical Cyclone Heat Potential (TCHP) was created. TCHP is a measure of the integrated vertical temperature between the sea surface temperature and the estimate of the depth of the 26°C isotherm. 26°C is used because 26°C is the magic temperature above which hurricanes form. The TCHP units are energy per surface area (typically kiloJoules/cm² where 1 kJ = 239 calories). The variation in the vertical thermal structure of the ocean is shown in the cartoon to the right. This material is taken from a NOAA Tropical Cyclone Heat Potential website.

Example TCHP calculation: Note the grey area on the upper right of the figure which closely reflects our example calculation here. Suppose the ocean temperature at the surface ("sea surface temperature" is SST) is 28°C and this temperature extends to 50 m depth. The ocean temperature then decreases linearly with depth and reaches 26°C at a depth of 100 meters. TCHP is defined using the temperature minus 26°C which is 2° from the surface to a depth of 50 m and then decreases linearly to 0° at 100 m depth.

Approximate energy available to the hurricane

Energy available ~ (temperature-26°C) x heat capacity of water x water density x depth of warm layer

Energy available in the first 50 m is 2 degrees x 1 calorie/degree/gram(water) x 1 gram(water)/cm³ x 50 m x 100 cm/m = 10,000 cal/cm²
In the 50 m to 100m depth interval, the average temperature is 27°C so
the Energy available in the 50 m to 100 m depth interval is 1 degree x 1 calorie/degree/gram(water) x 1 gram(water)/cm³ x 50 m x 100 cm/m = 5,000 cal/cm²
So the energy available is 15,000 cal/cm² = 15 kcal/cm² = 63 kJ/cm²

Note that the TCHP depends on both the warm near-surface temperature and depth to which this warm water extends

The TCHP is estimated from satellite data. Key satellite variables are the SST and the height of the ocean. Warming the ocean causes it to expand slightly which causes its height to increase. The height is measured by orbiting altimeters. The figure below shows the TCHP estimated for the Gulf of Mexico at the time of hurricane Rita. Notice how closely the TCHP is related to the intensity of Hurricane Rita. It appears that the sharp intensification of Rita over the middle of the Gulf was related to Rita passing over a region of high TCHP. The decrease in Rita's strength as it neared the Texas/Louisiana coast was apparently related to its passing over a region containing less energy (TCHP) in the ocean surface layer in comparison with that in the middle of the Gulf of Mexico.

Figure:Tropical Cyclone Heat Potential and Rita's path and intensity. Tropical Cyclone Heat Potential field in the Gulf of Mexico during September 22, 2005. The path of Hurricane Rita is indicated with circles spaced every 3 hours with their size and color representing intensity (see legend). This hurricane intensified to category 5 as it traveled over the Loop Current and a warm core eddy (the finger of red and yellow). Rita diminished to category 3 as its path went over a region of lower TCHP (and cooler waters) outside the Loop Current and ring. The diamonds indicate the National Hurricane Center predicted track and intensity as it makes landfall, and are spaced by 24 hours. Altimeter data on NASA's Jason-1, the US Navy's GFO, and the European Envisat satellites provide sea surface height data used in generating the TCHP fields. from NASA Rita webpage

Heating of the atmosphere by a hurricane

Just as evaporation cools the ocean, the latent heat release into the atmosphere by the water vapor condensation warms the atmosphere. We estimate the atmospheric warming in a manner analogous to the ocean cooling. The heat capacity of air is 0.24 cal/degree/gram(air). The mass of atmosphere in a column is given by the surface pressure divided by gravity. 1000 mb is equivalent to an atmospheric column mass of 1000 grams per square cm.

Rearranging and solving for the increase in air temperature gives

Plugging in numbers using the average latent heat Katrina released into a column of 9000 calories for each square centimeter, yields an air temperature increase of
9000 cal/cm^2 / 0.24 cal/degree/gram(air) / 1000 g/cm² = 36°C.
Now, the air temperature does not actually increase by this much because as the air is warmed it becomes buoyant and rises and flows out from the top of the hurricane horizontally. As a result the air column is replaced at least twice as the hurricane passes by (based on the rainfall being roughly twice the water vapor the air column can hold). Therefore the rise in air column temperature in the hurricane is no more than half the amount above or <18°C. In terms of absolute temperature in Kelvin which is degrees Celsius plus 273.15, a typical hurricane surface air temperature of 27°C is 300K in absolute temperature. This increase in the absolute temperature of the air column can approach 10% near the center of the hurricane. The tremendous decrease in density associated with this warming is responsible for much of the ~10% decrease in surface pressure at the center of a 900 mb storm like Katrina and Rita.

Seasonal forecasting of Hurricanes

Several factors are considered in providing seasonal forecasts of hurricane numbers, intensities and number of landfalling hurricanes. Among the main ones are forecasts of SST and wind shear in the hurricane regions. El Nino and La Nina substantially influence the number of hurricanes in the north Atlantic and northeastern Pacific.

The influence of El Niņo and La Nina on the Atlantic and Pacific hurricane seasons

As discussed previously, hurricanes have trouble forming and intensifying in the presence of wind shear. Vertical wind shear refers to the change in winds with height. Hurricanes require low vertical wind shear (less than 8 ms-1). Dr. Gray at the Colorado State University found that El Niņo and La Niņa affect the hurricane season. Specifically El Niņo contributes to more eastern Pacific hurricanes and inhibits Atlantic hurricanes while La Niņa does just the opposite. The influence of El Niņo is particularly evident in the relative low number of Atlantic hurricanes in the strong El Nino years of 1983 and 1997.

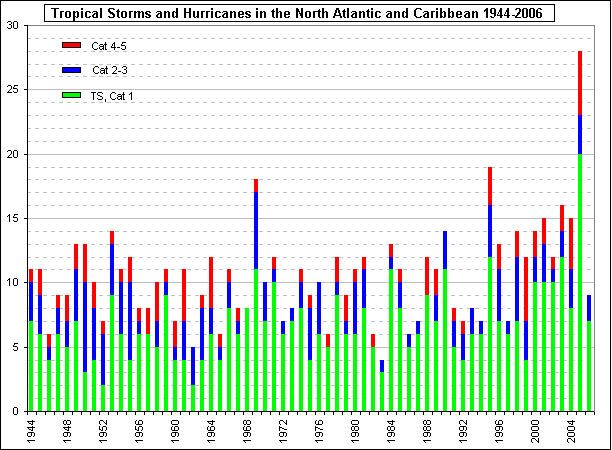Figure 9: The number of hurricanes (separated by category) in the Atlantic basin each year from 1944 to 2006.

SAHARAN DUST?